First Order Pin Joints: This type of joints have two or more Links and are said to be of Order One less than the Number of Links joined.
In the above Fig. you can notice there are two links joined by a pin, therefore, the Order of the joint is First. The Degree of Freedom i.e. DOF = One
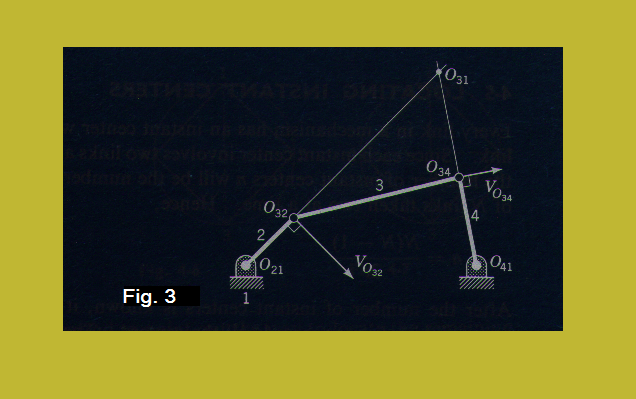
Second Order Pin Joint: In this type of joint there are there links joined by a pin. Since the number of Links is three the Order (of the joint will be one less than three) is said to be Second Order.
In the Fig., posted above, you can see if Link L1 is taken as the reference Link then the other two Links L2 and L3 will rotate, (with respect to L1 as shown in the Fig.) the Degree of Freedom i.e. DOF = Two
A Roller on A Plane: This is an example of Higher pair. The pair is entirely different type of pair (joint). You can see in the Fig. posted below, that the Roller is simply placed on a plane without being joined by a pin or a slot, but is in contact with the plane.
There are three kinds of possible motions in such joints (pairs), the Roller can simply Roll Or can Slide Or can Roll and Slide, over the plane depending on the friction between roller and the plane. The DOF is either One or Two. When the Roller only rolls the DOF = 1, when it only slides DOF = 1 and when it rolls and slide at the same time the DOF = 2.
In the above Fig. you can notice there are two links joined by a pin, therefore, the Order of the joint is First. The Degree of Freedom i.e. DOF = One
Second Order Pin Joint: In this type of joint there are there links joined by a pin. Since the number of Links is three the Order (of the joint will be one less than three) is said to be Second Order.
In the Fig., posted above, you can see if Link L1 is taken as the reference Link then the other two Links L2 and L3 will rotate, (with respect to L1 as shown in the Fig.) the Degree of Freedom i.e. DOF = Two
A Roller on A Plane: This is an example of Higher pair. The pair is entirely different type of pair (joint). You can see in the Fig. posted below, that the Roller is simply placed on a plane without being joined by a pin or a slot, but is in contact with the plane.
There are three kinds of possible motions in such joints (pairs), the Roller can simply Roll Or can Slide Or can Roll and Slide, over the plane depending on the friction between roller and the plane. The DOF is either One or Two. When the Roller only rolls the DOF = 1, when it only slides DOF = 1 and when it rolls and slide at the same time the DOF = 2.